If 1=5, 2=25, 3=325, 4=4325 Then 5 = ?
Fun Math: 6
Find If 1=5, 2=25, 3=325, 4=4325 Then 5 = ? check it!
This interesting puzzle presents a unique pattern to decipher: If 1=5, 2=25, 3=325, 4=4325, then what does 5 equal? At first glance, it may seem challenging, but a closer look reveals the solution.
Let’s analyze the given equations:
- 1 = 5
- 2 = 25
- 3 = 325
- 4 = 4325
It appears that the pattern involves a specific relationship between the numbers and their outputs. However, the key to solving this puzzle lies in understanding that the pattern is cyclical. Notice the first equation, 1 = 5. The puzzle is hinting at a reverse relationship, indicating that the equations are meant to be reversed at the end.
Given this cyclical pattern, if 1 equals 5, then by the same logic, 5 would revert back to 1.
Therefore, the answer to the puzzle is: 5 = 1
This puzzle is an excellent exercise in recognizing patterns and understanding the cyclical nature of the relationship presented. It challenges our perception and encourages us to think beyond conventional patterns, making it a great brain teaser to share and discuss with friends.
Like, Share, Comment and Subscribe!

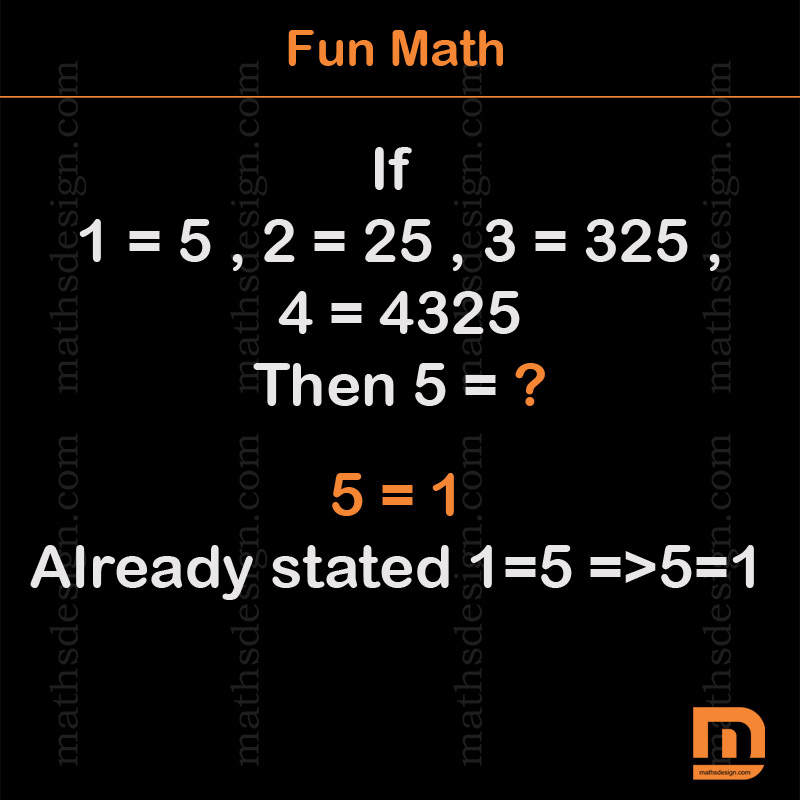



Recent Comments