Logarithm table
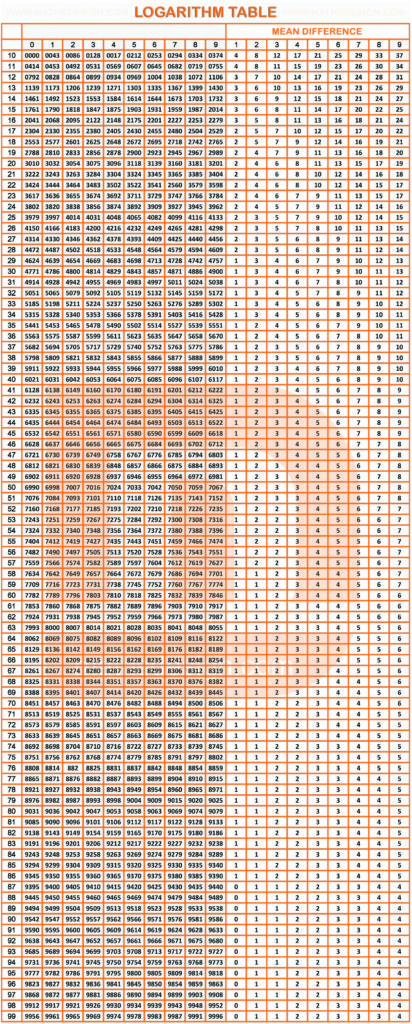
Before computers and calculators, logarithms were quickly calculated using a logarithm table. These tables are still useful for quickly calculating logarithms or multiplying large numbers, once you figure out how to use them.
Definition:
In mathematics, the logarithm is the inverse function of exponentiation. That means the logarithm of a number x to the base b is the exponent to which b must be raised, to produce x.
A logarithm is a mathematical function that represents the exponent to which a given base must be raised to obtain a specific number. In other words, if you have a logarithm with a base “b” and a number “x”, then the logarithm of “x” to the base “b” is denoted as “log_b(x)”, and it is defined by the equation:
x = b^(log_b(x))
Here, “x” is the number for which you want to find the logarithm, and “b” is the base of the logarithm. The logarithm essentially provides a way to “undo” exponentiation and convert it into a simpler form of mathematical operation.
Logarithms have various properties that make them useful in a wide range of mathematical and scientific applications:
The inverse of Exponentiation: Logarithms are the inverse operations of exponentiation. They allow you to solve for an exponent when you know the base and the result of the exponentiation.
Logarithmic Scale: Logarithmic scales used to represent exponential growth or large ranges of values. For example, the Richter scale for measuring earthquake magnitudes uses a logarithmic scale.
Simplifying Complex Operations: Logarithms can simplify mathematical operations involving multiplication, division, and exponentiation, turning them into addition, subtraction, and multiplication, respectively.
Solving Equations: Logarithms can help solve equations involving exponential terms. As they allow you to isolate the variable from the exponent.
Rates of Growth: In calculus, logarithms used to analyze and compare rates of growth of different functions.
Number Theory: Logarithms used in number theory to study properties of prime numbers and other mathematical structures.
Common logarithms (base 10) and natural logarithms (base “e”, where “e” is the mathematical constant approximately equal to 2.71828) the most frequently used types of logarithms. The natural logarithm is particularly important in calculus, complex analysis, and various scientific and engineering fields.
Logarithms played a significant role in the history of mathematics. Especially before the advent of calculators and computers. As they allowed for easier computation of complex calculations. However, in modern times, with the availability of advanced computational tools. Logarithms often used more for their mathematical properties and theoretical applications than for practical calculation.

Recent Comments